
Return to Basic Equipment Menu
|
Protractors
In practice, fields of vision are described in circles
and cones which are broken down into 360 degrees. Most
artistic endeavors contain an inherent fudge factor,
allowing the artist to place lines where they look right
merely by "guessing" where they look best. The human brain
is very forgiving when angles and depth cues are involved.
Sometimes more precision is warranted, and angles must be
calculated precisely. A protractor is used to both measure
and create proper angles. If you have ever had the occasion
to use polar coordinates, then you are already familiar with
this sort of device.
|
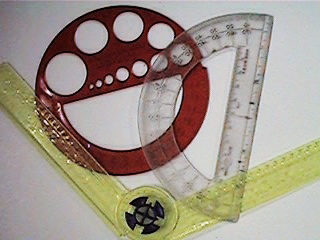
Some protractors measure a full 360 degrees, but most stop at 180
degrees and have one straight flat side. Since a full circle is equal
to 360 degrees, the exercising of a little basic algebra makes a full
circle protractor unnecessary. Since they allow for the measurement
and creation of angles in tighter and smaller circumstances, swing
arm protractors are also very handy.

Angles are measured from the "station point" which should be made
evident in the design of the protractor. Sometimes this is a hole in
the device which is meant to center over the intersection of an
angle, sometimes it is just an obvious marking along the edge.
Measurements taken from other than the station point will be
incorrect.
Angle Measurements of Regular
Polygons
A regular polygon is any shape consisting of straight sides and
angles where each side has an equal length and each angle has an
equal degree. Thus a square is a regular polygon because each corner
is a 90 degree angle, and each side measures the same length. A
rectangle is not because all of its sides need not measure the
same.

Sometimes it is necessary to measure out specific shapes when
drawing, especially when a specific object is to be rendered in
linear perspective. To calculate the angle measures of a regular
polygon first count the number of corners (vertices). Next subtract
two and then multiply the result by 180 degrees. Finally divide the
product by the original number of corners.
(number of corners - 2) *180/number
of corners
Thus a regular pentagon (five sides) has an angle measure of 108
degrees for each internal angle. [(5-2)*180/5]
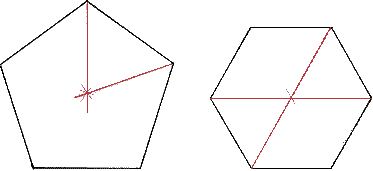
To find the center of a regular polygon with an odd number of
sides divide the measurement of one corner in half and draw a line
from that corner to the other side. Do the same with another corner.
The lines will cross at the center of the shape. The same method will
work for a polygon with an equal number of sides, but it is simpler
just to connect opposite corners.
Regular polyhedrons are 3 dimensional shapes constructed of the
regular polygons. There are five regular polyhedrons which are known
as the platonic solids.
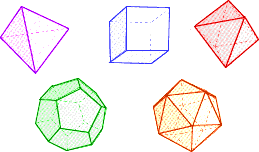
Plato theorized that everything in nature was made from smaller
particles, sort of like atoms. He reasoned that these smaller
particles must be regular polyhedrons like those pictured above. His
concept was based on the classical elements, so his theory may seem
strange to our periodic table mentality.
|
# of sides
|
Name
|
Element
|
|
4
|
tetrahedron
|
Fire
|
|
6
|
hexahedron (cube)
|
Earth
|
|
8
|
octahedron
|
Water
|
|
12
|
dodecahedron
|
Cosmos
|
|
20
|
icosahedron
|
Air
|
|Templates|Triangles|Curves|Rulers|Protractors|Lettering
Guides|Compasses|
[Return
to top of page]





